Allysa's scribepost for November 4, 2010

We were all given a blog post again today. I was assigned question 22, on the unit 2.2. This question looked hard at first, but when you look at it again, its real simple and straight forward.

22. Replace each blank square with <, >, or = to make each statement true.













a) I started with converting my fractions into decimals.


Now I know they're equal, because at the top, they have the same numbers. That is where you find your
decimal.








b) The other number is -0.6 (Repeating) as shown in the diagram at the top. -3/5 or -0.6 is greater because its a negative.





c) This one is pretty straight forward. -1 3/10 is equivalent to - (-13/-10) because they're both the same as saying -13/10 as an improper fraction.


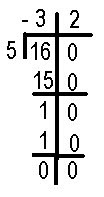
d) The other number is -3.25. -3.25 is less than -3.2 because it is negative so the smaller number is larger.









e) This is a more complicated one. - 8/12 is larger. The reason why the decimal numbers are random is because for the first one, I divided 12 by 3 to get 4. Now you have to do that for the percentage. 100% divided by 3 is 33(repeating)%. Since you need 8, you multiply the 4 by 2. When you do that, you have to multiply the percentage by 2. You now should have 66(repeating)%.
**This applies to the next 2 questions also.**















f) I prefer converting it into an improper fraction. - 17/6 is greater because when I converted them into decimals, since it was negative, the smaller number was greater.
For the second conversion, instead of dividing the last number into 3, I divided it into 4. So, 8 divided by 4 is 2. 100% divided by 4 is 25%. 2 multiplied by 3 is 6. Therefore, 25% multiplied by 3 is 75%.














**NOTE: Don't let the negatives trick you. If it is a negative, the smaller number will always be greater. Also if one number in a fraction is a negative, the whole number is.**

Thank you for going through my blog. Please leave a comment behind, or any advice on what I did wrong.